题目描述

原题链接

给定一个二叉树的根节点 root ，返回它的 中序 遍历。

示例 1：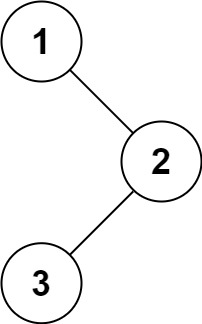

输入：root = [1,null,2,3]
输出：[1,3,2]

示例 2：
输入：root = []
输出：[]

示例 3：
输入：root = [1]
输出：[1]

示例 4：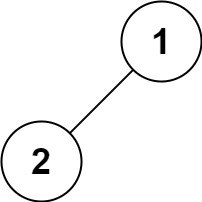

输入：root = [1,2]
输出：[2,1]

示例 5：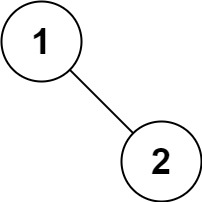

输入：root = [1,null,2]
输出：[1,2]

提示：

• 树中节点数目在范围 [0, 100] 内
• -100 <= Node.val <= 100

进阶: 递归算法很简单，你可以通过迭代算法完成吗？

个人解法

Javascript

递归

/*
 * @lc app=leetcode.cn id=94 lang=javascript
 *
 * [94] 二叉树的中序遍历
 */
// @lc code=start
/**
 * Definition for a binary tree node.
 * function TreeNode(val, left, right) {
 *     this.val = (val===undefined ? 0 : val)
 *     this.left = (left===undefined ? null : left)
 *     this.right = (right===undefined ? null : right)
 * }
 */
/**
 * @param {TreeNode} root
 * @return {number[]}
 */
var inorderTraversal = function (root) {
  const result = [];
  var mid = function (root) {
    if (root == null) {
      return;
    }
    mid(root.left);
    result.push(root.val);
    mid(root.right);
  }
  mid(root);
  return result;
};
// @lc code=end

Java

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode() {}
 *     TreeNode(int val) { this.val = val; }
 *     TreeNode(int val, TreeNode left, TreeNode right) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
class Solution {
    List<Integer> tree=new ArrayList<>();
    public List<Integer> inorderTraversal(TreeNode root) {
        inorderTraversal(root,1);
        return tree;
    }
    public void inorderTraversal(TreeNode root,int n) {
        if (root==null){
            return ;
        }
        inorderTraversal(root.left,0);
        tree.add(root.val);
        inorderTraversal(root.right,0);
    }
}

其他解法

Java

迭代

class Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        Deque<TreeNode> stk = new LinkedList<TreeNode>();
        while (root != null || !stk.isEmpty()) {
            //一直找到最左边的节点
            while (root != null) {
                stk.push(root);
                root = root.left;
            }
            root = stk.pop();
            res.add(root.val);
            //如果为左子树叶子节点，右子树为空（每次被找过的节点都可以视为不存在）
            //根节点还在栈中存着
            root = root.right;
        }
        return res;
    }
}

Morris 中序遍历

class Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<Integer>();
        TreeNode predecessor = null;
        while (root != null) {
            if (root.left != null) {
                // predecessor 节点就是当前 root 节点向左走一步，然后一直向右走至无法走为止
                predecessor = root.left;
                while (predecessor.right != null && predecessor.right != root) {
                    predecessor = predecessor.right;
                }
                // 让 predecessor 的右指针指向 root，继续遍历左子树
                if (predecessor.right == null) {
                    predecessor.right = root;
                    root = root.left;
                }
                // 说明左子树已经访问完了，我们需要断开链接
                else {
                    res.add(root.val);
                    predecessor.right = null;
                    root = root.right;
                }
            }
            // 如果没有左孩子，则直接访问右孩子
            else {
                res.add(root.val);
                root = root.right;
            }
        }
        return res;
    }
}

Javascript

迭代

/*
 * @lc app=leetcode.cn id=94 lang=javascript
 *
 * [94] 二叉树的中序遍历
 */
// @lc code=start
/**
 * Definition for a binary tree node.
 * function TreeNode(val, left, right) {
 *     this.val = (val===undefined ? 0 : val)
 *     this.left = (left===undefined ? null : left)
 *     this.right = (right===undefined ? null : right)
 * }
 */
/**
 * @param {TreeNode} root
 * @return {number[]}
 */
var inorderTraversal = function (root) {
  const res = [];
  const stk = [];
  while (root || stk.length) {
    while (root) {
      stk.push(root);
      root = root.left;
    }
    root = stk.pop();
    res.push(root.val);
    root = root.right;
  }
  return res;
};
// @lc code=end