Discrete Differential Geometry Lecture

image.png

The Mathematical Foundations of Manifold Learning

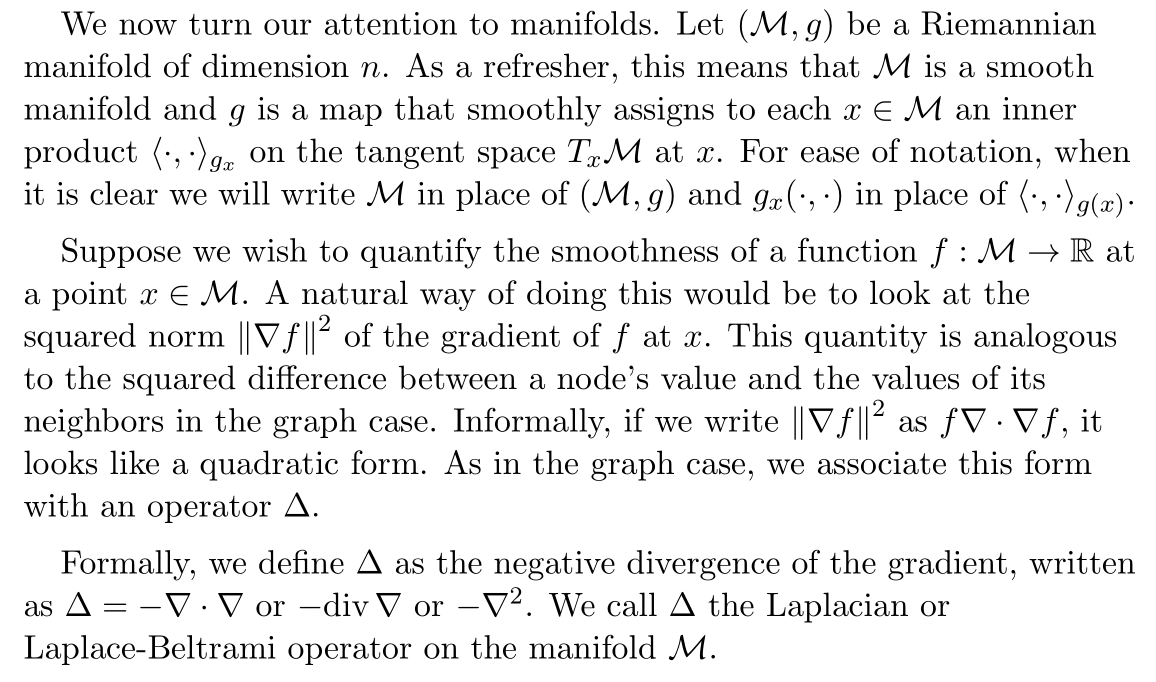
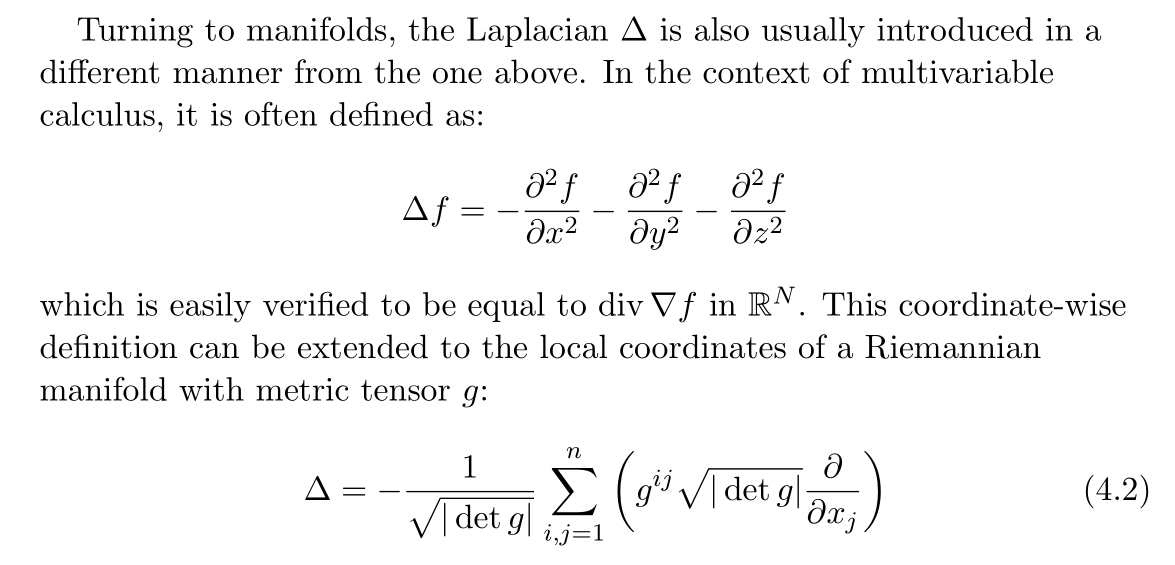
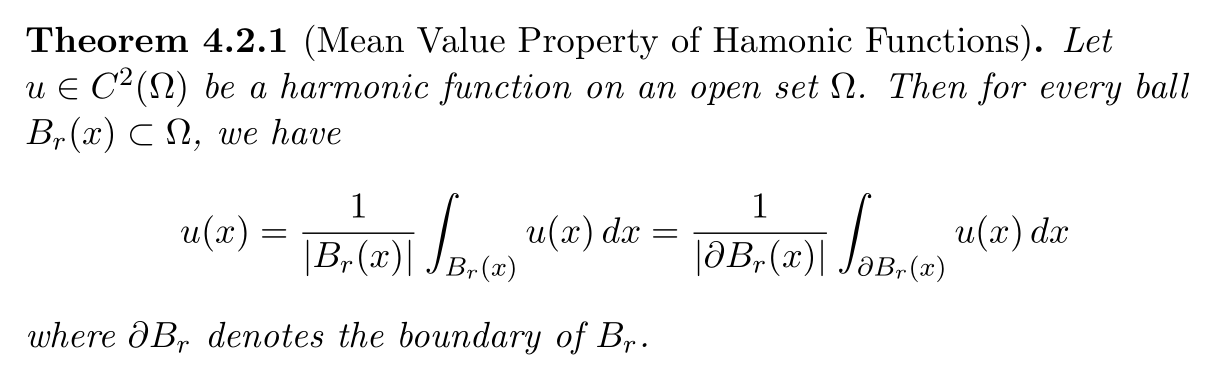
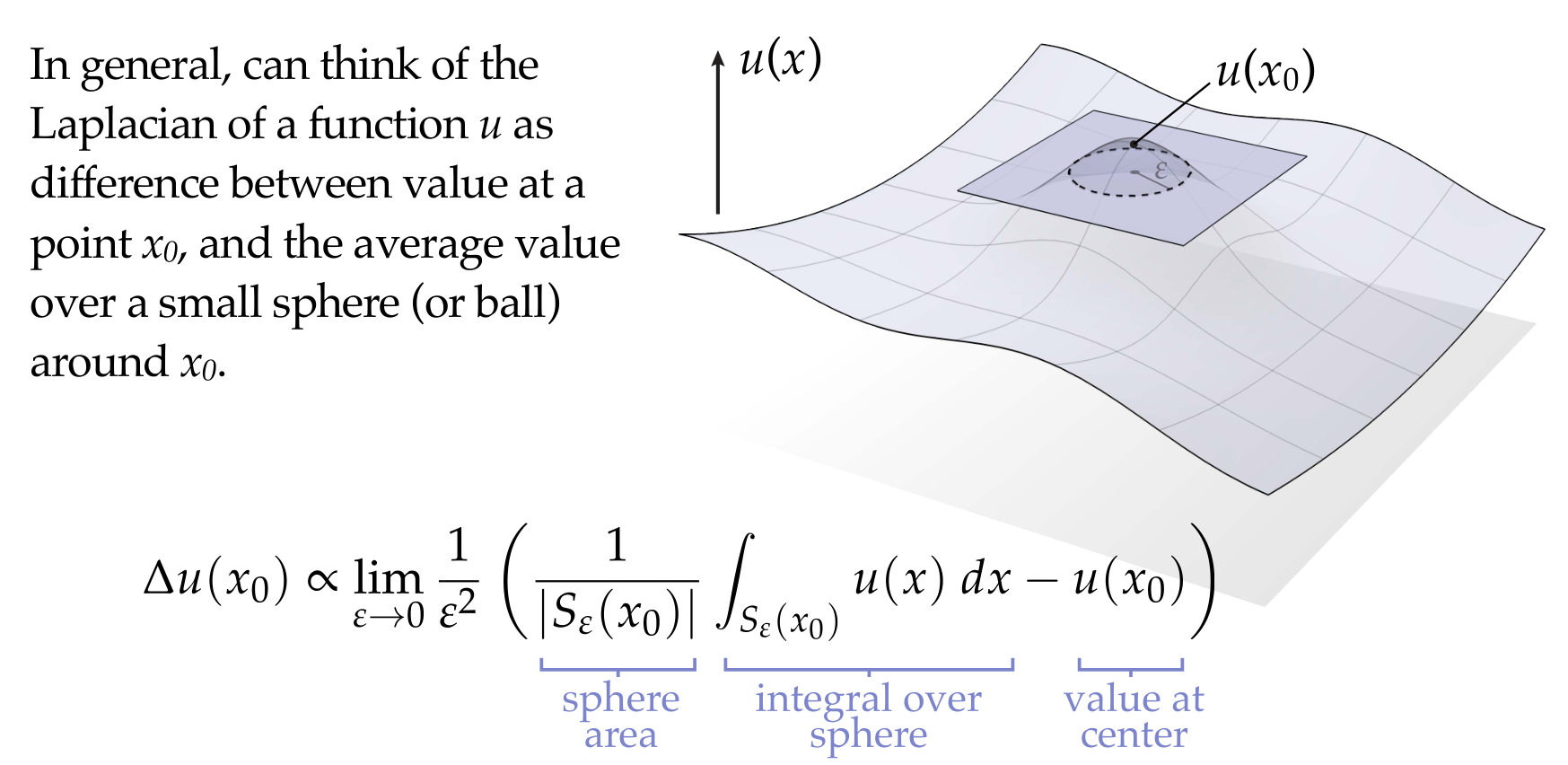
image.png
image.png

image.png
对于流形来说，如果 u 在任意球上的平均值等于球心处的值，那么称 u 是调和的。
对于图来说，就是 u 在一个顶点上的值等于任意 k-hop 邻域的平均值，也就是 u 在同一连通分支上的值都相等，写作 Lu=0，这样的 u 是调和的。

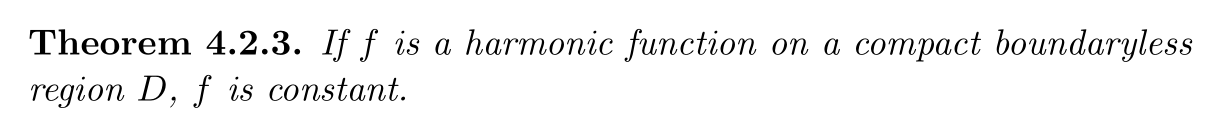
image.png
有限图上的调和函数 对应 无边界的紧流形（闭流形），后者是一个常数。

:::info We emphasize that finite graphs are analogous to closed (i.e. compact and boundaryless) manifolds, rather than those with boundary. :::

而有界区域上的调和函数，它的值由边界上的值决定：
image.png
由此可推出 极大值原理
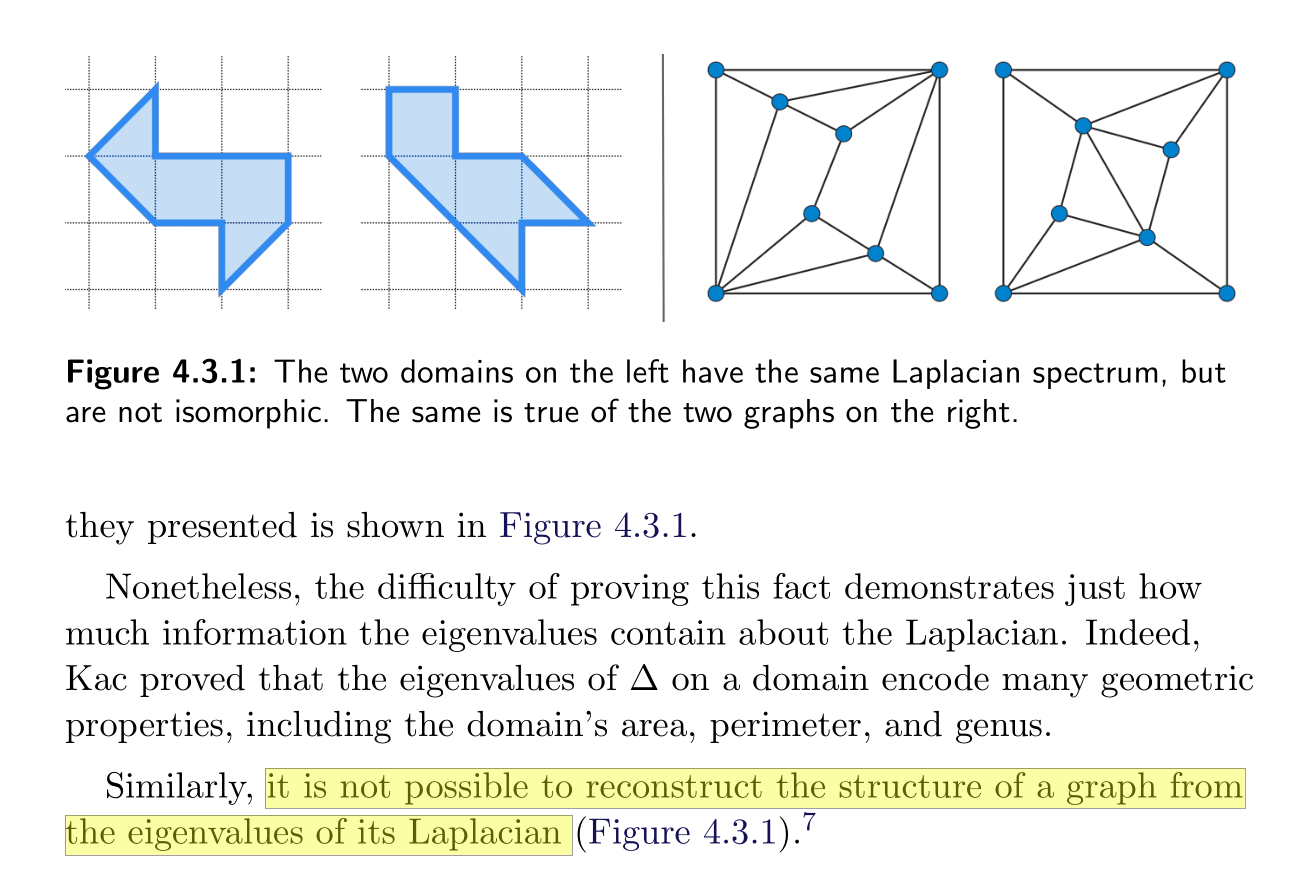
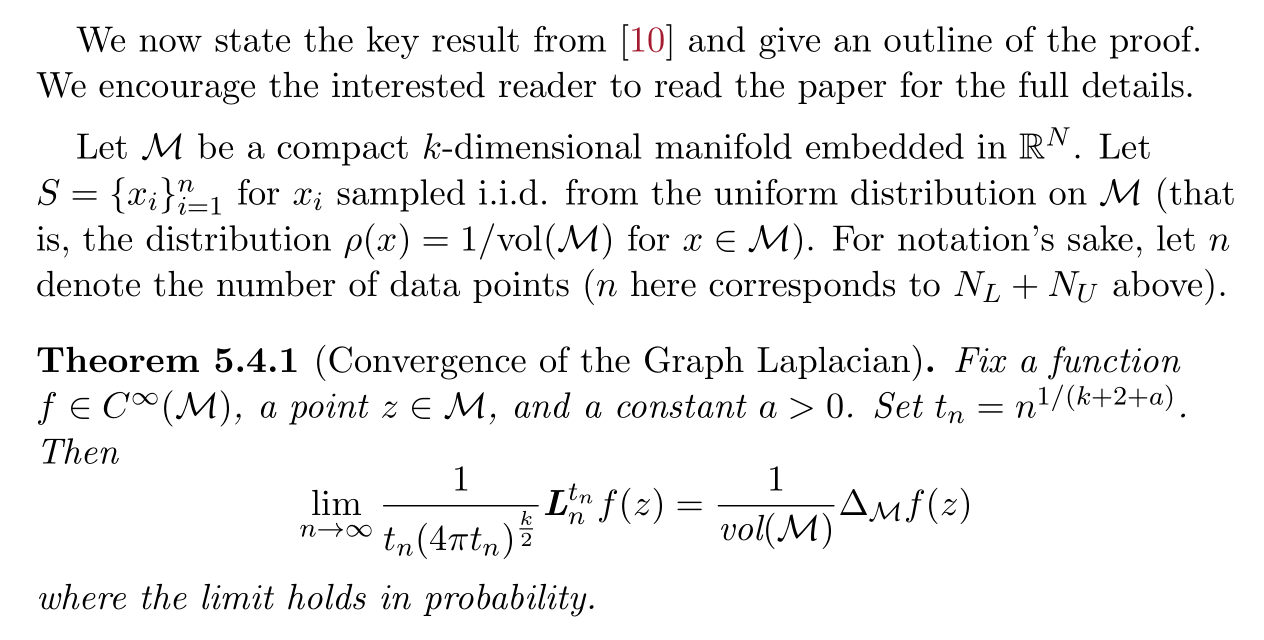
image.png

image.png

image.png
image.png
结论还是挺有意思的。