- SS16 Derivatives
- can be either deliverable or cash-settled contracts
#have contract prices set, zero value at initiation
#trade on organized exchanges
#future contracts are standardized
#clearhousing is the counterparty (low counterparty risk)
#government regulations exist
#minimum price fluctuation (tick size)/ daily price move limit/ settlement date/ trading times
#margin balance needed
settlement price: an average of prices of the trades during the last period of trading (closing period); On its final day of trading the settlement price is equal to the spot price of the underlying asset
long position: buyer of the futures contract
short position: seller of the futures contract
open interest: 未平仓合约 number of outstanding futures contracts of a specific kind
clearing house: splitting each trade once it is made and acting as the opposite side of each position, remove counterparty risks
margin: the money that must be deposited before entering into a future contract (no loan, no interest charge)
marking to market: Each day, the margin balance in a futures account is adjusted for any gains and losses in the value of the futures position based on the new settlement price
initial margin: the amount that must be deposited in a futures account before a trade may be made
maintenance margin: the minimum amount of margin that must be maintained in a futures account.
price limits: exchange-imposed limits on how each day’s settlement price can change from the previous day’s settlement price
limit move: 如果price move 超过price limits, no trades will take place
limit up; limit down; locked limit - R49 Basics of Derivative Pricing and Valuation
- a explain how the concepts of arbitrage, replication, and risk neutrality are used in pricing derivatives;
- b distinguish between value and price of forward and futures contracts;
- c calculate a forward price of an asset with zero, positive, or negative net cost of carry;
- d explain how the value and price of a forward contract are determined at expiration, during the life of the contract, and at initiation;
- e describe monetary and nonmonetary benefits and costs associated with holding the underlying asset and explain how they affect the value and price of a forward contract;
- f define a forward rate agreement and describe its uses;
- g explain why forward and futures prices differ;
- h explain how swap contracts are similar to but different from a series of forward contracts;
- i distinguish between the value and price of swaps;
- j explain the exercise value, time value, and moneyness of an option;
- k identify the factors that determine the value of an option and explain how each factor affects the value of an option;
- l explain put–call parity for European options;
- m explain put–call–forward parity for European options;
- n explain how the value of an option is determined using a one-period binomial model;
- o explain under which circumstances the values of European and American options differ.
SS16 Derivatives
R48 Derivative Markets and Instruments
a define a derivative and distinguish between exchange-traded and over-the counter derivatives;
- Define a derivative
A derivative is a security that derives its value from the value or return of another asset or security.
(perfect correlation with another asset’s reurn)
- exchange-traded and over-the-counter
Exchange-traded derivatives: standardized and backed by a clearinghouse
Over-the-counter: largely unregulated markets; with a counterparty; may expose to default risk
b contrast forward commitments with contingent claims;
- forward commitments
forward commitments: legally binding promise to perform some action in the future.
cate.: forward contracts, futures contracts, and swaps__
- contingent claims
contingent claims: claim (to a payoff) that depends on a particular event.
options: depend on a stock price at some future date
credit derivatives: depend on a credit event such as a default or ratings downgrade
c define forward contracts, futures contracts, options (calls and puts), swaps, and credit derivatives and compare their basic characteristics;
- forward contracts
甲 agrees to buy and 乙 to sell a physical or financial asset at a specific price on a specific date in the future
- no payment at initiation
forward price: the price specified in the forward contract
forward value:
long forward position: who agrees to buy the financial or physical __asset
short forward position: who agrees to sell or deliver__
cash-settled forward contract: pays cash at expiration { [forward price, market price] 的差额}
- futures contracts
standardized and exchange-traded forward contract
- characteristics
can be either deliverable or cash-settled contracts
#have contract prices set, zero value at initiation
#trade on organized exchanges
#future contracts are standardized
#clearhousing is the counterparty (low counterparty risk)
#government regulations exist
#minimum price fluctuation (tick size)/ daily price move limit/ settlement date/ trading times
#margin balance needed
settlement price: an average of prices of the trades during the last period of trading (closing period); On its final day of trading the settlement price is equal to the spot price of the underlying asset
long position: buyer of the futures contract
short position: seller of the futures contract
open interest: 未平仓合约 number of outstanding futures contracts of a specific kind
clearing house: splitting each trade once it is made and acting as the opposite side of each position, remove counterparty risks
margin: the money that must be deposited before entering into a future contract (no loan, no interest charge)
marking to market: Each day, the margin balance in a futures account is adjusted for any gains and losses in the value of the futures position based on the new settlement price
initial margin: the amount that must be deposited in a futures account before a trade may be made
maintenance margin: the minimum amount of margin that must be maintained in a futures account.
price limits: exchange-imposed limits on how each day’s settlement price can change from the previous day’s settlement price
limit move: 如果price move 超过price limits, no trades will take place
limit up; limit down; locked limit
- options (calls and puts)
An option contract gives its owner the right, but not the obligation, to either buy or sell an underlying asset at a given price
exercise price/strike price: the given price in the contracts
option premium: the price of an option
call option: the right to purchase the underlying asset at a specific price for a specified time period
put option: the right to sell the underlying asset at a specific price for a specified time period.
option writer: seller of an option (不一定是underlying的seller)
| long **call** | the buyer of a call option | the right to buy an underlying asset |
|---|---|---|
| short call | the writer (seller) of a call option | the obligation to sell the underlying asset |
| long **put** | the buyer of a put option | the right to sell the underlying asset |
| short put | the writer (seller) of a put option | the obligation to buy the underlying asset |
American options: may be exercised at any time up to and including the contract’s expiration date.
European options: can be exercised only on the contract’s expiration date
Bermuda option: contract that can only be exercised on predetermined dates.
- swaps 掉期
Swaps:agreements to exchange a series of payments on periodic settlement dates over a certain time period [事实上只交易净值]
- characteristics
NO payment at initiation custom instruments
NOT traded in any secondary market largely unregulated
default risk is important large institutions are main participants
plain vanilla interest rate swap: fixed-rate interest payment with floating-rate interest payment
[specified notional priciple amount]// fixed-rate payer & float-rate payer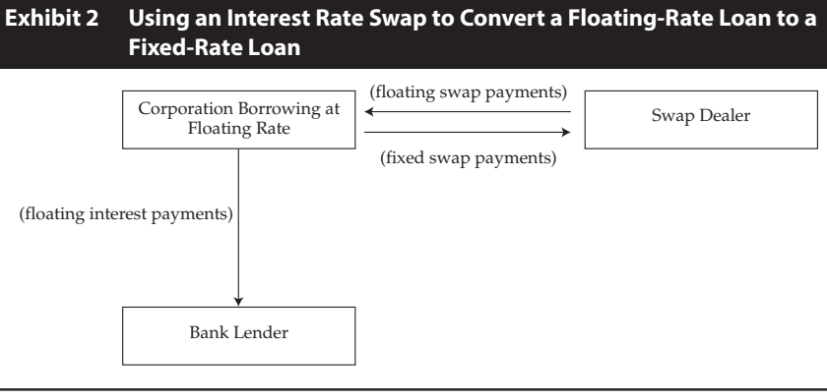
basis swap: trading one set of floating rate payments for another 基准掉期
notion principal: principal specified in the contract (no payment of principal in real) 名义本金不实际支付
- credit derivates
a contract that provides a bondholder (lender) with protection against a downgrade or a default by the borrower
credit default swap (CDS): A bondholder pays a series of cash flows to a credit protection seller and receives a payment if the bond issuer defaults. 本质上是对违约行为的一种保险,一般由债权人lender购买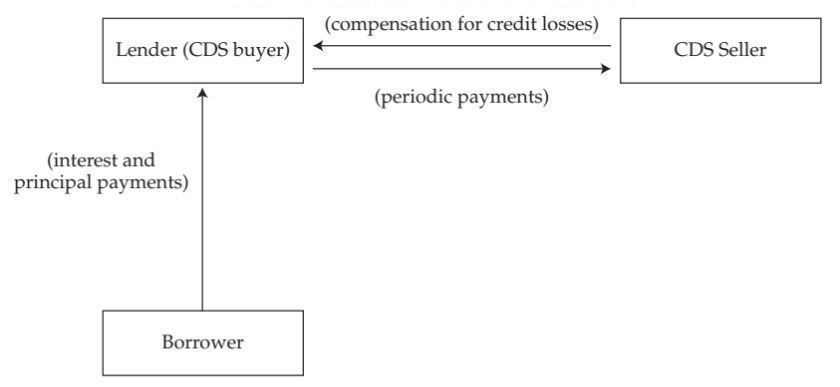
credit spread option: If the bond’s credit quality decreases, its yield spread will increase [future events happen] and the bondholder will collect a payoff on the option 信用利差期权,注意是给bondholder设计的
d determine the value at expiration and profit from a long or a short position in a call or put option;
| value at expiration | profit at expiration | |
|---|---|---|
| long call | max**(Pspot-**Pstrike,**0**) | -futures premium+max(Pspot-Pstrike,0) |
| short call | +futures premium-max(Pspot-Pstrike,0) | |
| long put | max**(**Pstrike-**Pspot,0**) | -futures premium+max(Pstrike-**Pspot,0)** |
| short put | +futures premium-max(Pstrike-**Pspot,0)** |
e describe purposes of, and controversies related to, derivative markets;
- purpose
- provide price information
- allow risk to be managed and shifted among market participants
- reduce transaction costs
controversies
arbitrage
Arbitrage: a return greater than the risk-free rate can be earned by holding a portfolio of assets that produces a certain (riskless) return. 回报率高于市场无风险利率,且具有确定性(无风险)的投资机会存在
- arbitrage’s role
- Arbitrage opportunities arise when assets are mispriced.
- 促进市场有效定价:Trading by arbitrageurs will continue until they affect supply and demand enough to bring asset prices to efficient (no-arbitrage) levels. 套利者进行交易→影响供求→价格向无套利水平移动
套利的两种理解思路↓**
- law of one price: Two portfolios with identical cash flows in the future (regardless of future events) should have the same price. 两项投资未来的现金流在任何情况下均一致,二者价格应相同
- If a portfolio of securities or assets will have a certain payoff (no risk) in the future, the return on the portfolio is the risk free rate. 如果某项资产未来有一确定收益,则其对应的收益率必为无风险收益率/两项无风险投资的收益率必须相等
R49 Basics of Derivative Pricing and Valuation
a explain how the concepts of arbitrage, replication, and risk neutrality are used in pricing derivatives;
- arbitrage
In contrast to valuing risky assets as the (risk-adjusted) present value of expected future cash flows, the valuation of derivative securities is based on a no-arbitrage condition
市场上的套利机会(不考虑交易成本)不会长期存在**,故而可以认为无套利机会是一种稳定状态,可以被用来对衍生品定价。**
衍生品的风险完全依赖于其标的资产(corr=1),所以可以通过构造衍生品和标的资产的投资组合,使其具有一个无风险收益(收益具有确定性),进而其收益率必须为无风险收益率(无套利定价): 合约定价(T时刻资产价格)
:资产即期价格(0时刻资产价格)
理解方式:
[1] 确定性收益
0时刻无风险借入S0,买入资产,进入远期短头寸,在T时刻交付资产,收入F0(T)
[2] 一价定律
a. 0时刻S0买入资产,持有到T时刻
b.0时刻进入远期长头寸,合约价格F0(T),买入T时刻到期的零息债券, par value=F0(T)
两者产生的现金流相同
risk-neutral pricing**: 套利定价的过程未考虑投资者的风险厌恶(no “risk premium method”) 故称为风险中性定价
另一种理解方式:套利定价的过程中构建了无风险的投资组合,所以其收益必须为无风险收益,而与个人投资者的风险厌恶程度无关
- replication
replication: replicating the payoffs on one asset or portfolio with those of a different asset or portfolio
例:
- risk neutrality
risk-averse: investor require a positive premium (higher return) on risky assets
risk-neutral: investors require no risk premium→discount expected FV of an asset or future cash flows at risk-free rate
b distinguish between value and price of forward and futures contracts;
- the value of futures and forward contracts is zero at initiation when the forward price is its no-arbitrage value.
- As the price of the underlying asset changes during the life of the contract, the value of a futures or forward contract position may increase or decrease.
price 是合约中规定的价格,也是远期/期货定价中的价格
value 可理解为对应时刻的交易损失/收益
c calculate a forward price of an asset with zero, positive, or negative net cost of carry;
net cost of carry : = PV(benefits of holding the asset) – PV(costs ofholding the asset)
benifit相当于降低了现货价格,cost相当于增加了现货价格,注意benefit和cost都需要进行折现,且当net cost为正时,benfit大于cost
d explain how the value and price of a forward contract are determined at expiration, during the life of the contract, and at initiation;
| value | contract price | |
|---|---|---|
| initiation | ||
| during the contract life | ||
| expiration |
其实valuate at initiation也可以使用公式,t=0代入,则value=0
e describe monetary and nonmonetary benefits and costs associated with holding the underlying asset and explain how they affect the value and price of a forward contract;
storage, insurance cost, dividend payment
convenience yield: nonmonetary benefits of holding an asset
这里相同资产可以理解为:现货要顺利成为T时刻的期货,期间可能需要支付各项成本,同时也获得某些好处,这是现货和期货有差异的地方,所以以money衡量,【现货+持有现货的成本-持有现货的好处】再进行TVM计算才等于【期货】
f define a forward rate agreement and describe its uses;
forward rate agreement (FRA): future interest rate is the underlying
The point of entering into an FRA is to lock in a certain interest rate for borrowing or lending at some future date.
One party will pay the other party the difference (based on an agreed-upon notional contract value) between the fixed interest rate specified in the FRA and the market interest rate at contract settlement.
long position in FRA: pay fixed, receive floating (大概的一个原则就是long一般获得将不确定性转化为确定性的好处)
uses: hedge the risk of borrowing and lending planed in the future
借款人:进long position,支出固息,收到浮息,用浮息还款。对冲利率上行风险(会使利息支付上升)
出借方:进short position,支出浮息,收到固息,固息作为出借本金的利息。对冲浮动利率下行风险(收到浮息减少)
Note a perfect hedge means not only that
the firm’s borrowing costs will not be higher if rates ↑, but also that the firm’s borrowing costs will not be lower if interest rates ↓
Return from both the short FRA and loaning the funds is the no-arbitrage rate that is the price of the FRA at initiation.
进入FRA短头寸(收到固定息,支出浮动息),并成为债权人(收到浮动息)=收到固定息⋙FRA之价格 at initiation
synthetic FRA: a bank can create the same payment structure with two LIBOR loans
(a) 在30~90天之间借款,利率为Libor (支出浮动息); 同时进入FRA的long position (收到浮动息,支出固定息)
最终效果:以固定利息,在30~90天间借款。固定息为FRA的contract rate
(b) 在即期市场借入120天Libor贷款,借出30天Libor贷款。最终效果:以固定息,在在30天~90天借款。
固定息为根据即期市场上的30天Libor和120天Libor计算出的利率
二者的最终payoff相同,根据无套利原则,二者对应的固定息应该相同
Forwards and futures serve the same function in gaining exposure to or hedging specific risks, but differ in their degree of standardization, liquidity, and, in many instances, counterparty risk.
g explain why forward and futures prices differ;
相同点:gain exposure; hedge specific risks
不同点:standardization; liquidity;… ; counterparty risk; 期货的逐日盯市原则(远期不需要提前支付anything)
———————————————————————————————————————————————-
逐日盯市** **(注:并不是price differ的主要原因)
gain and losses are settled each day and the margin balance is adjusted accordingly
margin账户中超过initial margin level的部分可以被取出
margin账户余额小于minimum margin level泽旭补齐至initial margin level
——————————————————————————————————————————-
- 利率不变/利率与期货价格不相关→远期与期货价格相同
- 期货价格与利率正相关:期货价格高于远期价格
利率上升→价格上升→excess margin上升→likely to earn more
利率下降→价格下降→但此背景下的资金机会成本较小
- 期货价格与利率负相关:期货价格低于远期价格
利率上升→价格下降→excess margin下降→likely cost more(高利率→高融资成本)
利率下降→价格上升→excess margin上升→再投资风险
h explain how swap contracts are similar to but different from a series of forward contracts;
A 1-year swap with quarterly payments, one party paying a fixed rate and the other a floating rate of 90-day LIBOR
1st payment (90 days from now) = S** - F** which is known at time zero
2nd payment (180 days from now) equivalent to a long position in an FRA with contract rate F that settles in 180 days and pays S**2 - F**2.
3rd payment (270 days from now) is equivalent to a long position in an FRA with contract rate F that settles in 270 days and pays S**3 - F**3.
4th payment (360 days from now) is equivalent to a long position in an FRA with contract rate F that settles in 360 days and pays S**4 - F**4**.
off-market forward: when a forward contract is created with a contract rate that gives it a non-zero value at initiation
i distinguish between the value and price of swaps;
price: the fixed rate of interest specified in the swap contract (the contract rate)
value: depends on how expected future floating rates change over time.
zero swap value at initiation
expected short-term future rates ↑,long position (fixed payer) has positive value
*expected short-term future rates ↓,long position (fixed payer) has negative value
j explain the exercise value, time value, and moneyness of an option;
Moneyness: refers to whether an option is in the money or out of the money
| CALL | In-the-money call | *long position 受益→in *long position 损失→out *无损益→at_ |
|
|---|---|---|---|
| Out-of-the-money call | |||
| at-the-money call | |||
| PUT | In-the-money put | ||
| Out-of-the-money put | |||
| at-the-money put |
intrinsic value/ exercise value:the maximum of zero and the amount that the option is in the money
time value: the amount by which the option premium (price) exceeds the intrinsic value
k identify the factors that determine the value of an option and explain how each factor affects the value of an option;
- price of the underlying asset
标的资产价格越高→期权内在价值(高的可能性)越高
- exercise price
执行价格X越高,看涨期权价格越低(in the money概率越低)
执行价格X越低,看跌期权价格越高(in the money概率越高)
- risk-free rate of interest
R**f上升,call option 价值上升
R**f上升,put option 价值下降
- volatility of the underlying
波动性越高,看涨/看跌期权价值均越高(期权就是从价格的波动性中得到的价值)
- time to experation
call option: 时间越长→波动性越高→价值越大
put option: 大部分put option同理 (部分欧式期权例外)
- cost and benefits of holding the asset
持有成本和持有收益影响标的资产现货和期货价格差,进而影响执行价格和期货价格的差,故而影响期权价格。
持有成本增加期货价格,进而看涨期权价格上升,看跌期权价格下降
持有收益降低期货价格,进而看涨期权价格下降,看跌期权价格上升
l explain put–call parity for European options;
put-call parity for European options is based on the payoffs of two portfolio combinations: a fiduciary call and a protective put
fiduciary call: long **call** (exercise price X) +pure-discount bond riskless, pays X at maturity
payoff at expiration:
protective put: share of stock+lo**ng put** (exercise price X)
payoff at expiration:
两者的pay off at expiration相等,故根据无套利定价原则,二者在0时刻价格相等
*c /欧式看涨期权价格 *X /合约执行价格(无风险零息债券面值) *R /无风险利率
*S /0时刻标的股票价格 *p /欧式看跌期权价格 *T /合约期限,put,call一样
Note: 基于公式,可以利用四种资产中的三种**合成**另外一种(即产生相同现金流)
m explain put–call–forward parity for European options;
将期权平价关系式中的股票资产S置换为远期资产,合约价格为。
在**0**时刻,远期资产的价值为,用以置换期权平价关系式中的
⋙
n explain how the value of an option is determined using a one-period binomial model;
U: size of up-move. D: size of down-move.
π: probability of an up-move. πD: probability of a down-move.
These two probabilities are NOT the actual probability of an up- or down-move. They are risk-neutral pseudo probabilities
| Calculation Step | ||
|---|---|---|
| 1 | 根据U/D计算D/U | |
| 2 | 计算π和πD | |
| 3 | 计算S**U和S**D | |
| 4 | 计算对应时刻,对应情景的期权价值ccT,D ;pT,U , pT,D | |
| 5 | 计算T时刻期权合约价值的期望E(c**T), E(pT**) | |
| 6 | 以无风险利率折现,得cp |
o explain under which circumstances the values of European and American options differ.
The prices of European and American options will be equal unless the right to exercise prior to expiration has positive value
- call option
no cash flows during the life →no advantage to early exercise→美式=欧式
(即使处于充分实值状态,也可以通过出售期权而非行权,获得更多收益)?
cash flows during the life→early exercise can be valuable cash flow前行权,call价格不变,还有benefit→美式≥欧式
- put option
deep in the money(也就赚那么多了,行权还可获得剩余期限的无风险收益)→美式≥欧式

